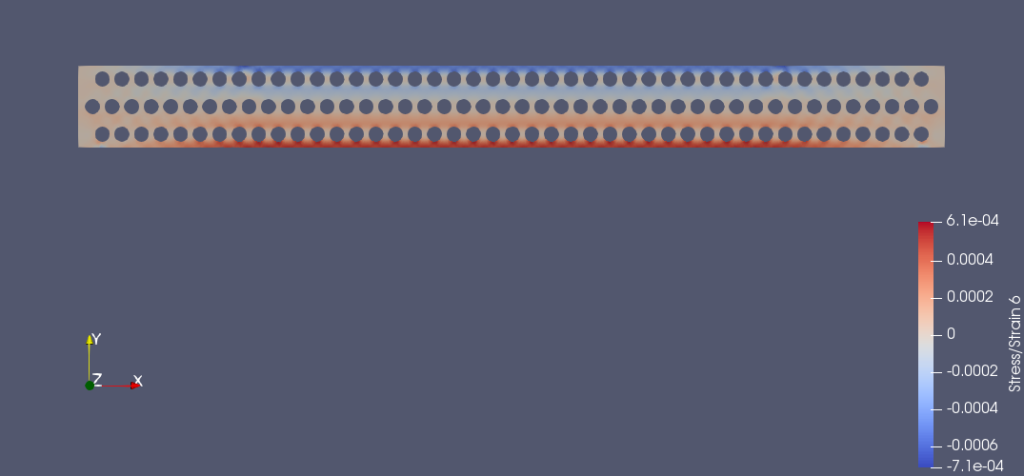
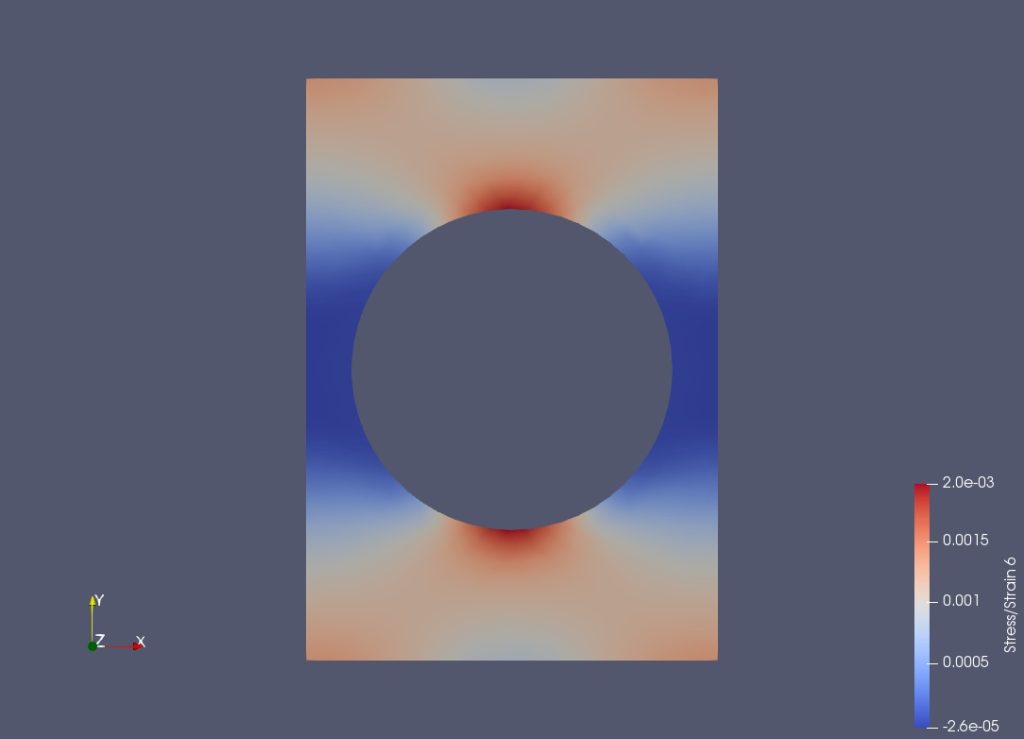
Under the scope of the project, one master thesis was written. Damjan Jurković, our Master’s student, wrote his Master’s thesis titled Pure bending in orthotropic micropolar elasticity. The thesis was written under the supervision of Prof. dr. sc. Gordan Jelenić and co-supervision of Doc. dr. sc. Sara Grbčić Erdelj and defended on 22 September 2023. As can be seen from the title of the area of interest was in the orthotropic micropolar continuum and the determination of its technical constants. The continuum’s properties were described with ten different technical constants: three Young’s moduli E, six Poisson’s ratios n and a characteristic bending length lb. Analytical expressions for stresses, strains and displacements for the case of the plane strain pure bending of this continuum were derived based on Gauthier’s expressions for the isotropic continuum. These expressions were then applied to the analysis of the aluminium specimens with artificially created microstructure, which are shown in the Experiments section. First, a unit cell of the LMD microstructure was defined as a representative sample of the material geometry. Four Poisson’s ratios were determined using virtual experiments via the FEM analysis in the FEAP software. After that, using the same methodology, pure bending virtual experiments were performed on aluminium specimens of sizes B1 to B4. Based on these results, homogenised Young’s moduli in the x-axis direction were determined for each of the specimens, while showing the size effect in the process. The results clearly showed that the height of the specimens is inversely proportional to their homogenised Young’s modulus (and thus to their stiffness). The homogenised Young’s moduli were regressed with a linear function and equated with the derived expression for the strain in the x-axis direction on the top/bottom of the aluminium beam. From here, Young’s modulus in the x-axis direction was determined using the Newton-Raphson method and from here characteristic bending length was also derived. By substituting these values into the beforehand derived expressions, rest of the technical constants were derived.
The thesis confirmed the value of the previously established methodological approach for determining the bending length of micropolar materials and implemented it to the orthotropic continuum. It also presented newly derived expressions for stresses, strains and displacements of this type of continuum. In future work, we will test this methodology on experimental data, which might answer the question whether one characteristic bending length is sufficient for the description of the orthotropic micropolar continuum.